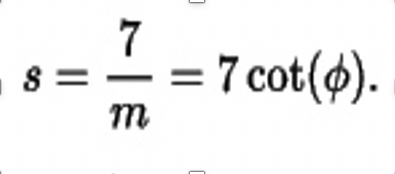A section of the Rhind Mathematical Papyrus, ca. 1550 BCE. It consists of reference tables, arithmetic, algebraic and geometric problems including volumes, and fractions (Courtesy of British Museum. London)
By Susanne L. Houfek –
The ancient Egyptians may not have taken trigonometry in school for entry level to a good university or even had a comprehensive education except for a select minority of the population, but they had a fairly complicated mathematical system, including algebra and geometry even if only a few select few understood higher mathematics including a fair amount of trigonometry. They had to be sufficiently numerate to count in multiples of at least 10 x 10 x 10 and to have an adequate numbering system with calculating fractions. Note the Old Kingdom Stele of Wepemnefret, Prince and a son of Khufu (Cheops), 4th Dynasty, at Giza (ca. 2500 BCE) immediately below. It shows numbers which reflect his offerings of 1000 pieces of linen, 1000 alabaster vessels, 1000 pieces of bread, 1000 jars of beer, 1000 antelopes and 1000 oxen to ease his way into the afterlife.

Stele of Wepemnefret, 4th Dynasty (Courtesy of Hearst Museum, University of California, Berkeley)
I may be dating myself here, but do you remember trig tables from your math courses? Remember the “opposite” and the “adjacent,” the sine, cosine and tangent, with their pesky inverses, the secant, the cosecant and the cotangent? You’re not alone if you’ve forgotten how all that works!
Wouldn’t it have been fantastic if Imhotep, Ankhhaf and all the other ancient Egyptian pyramid builders, architects, advisors, priests, quarrymen, team-leaders and stone-cutters had trig tables to look up the angle to cut their stones?
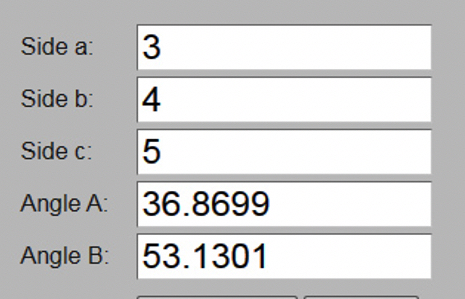
To understand what they might have done, we need the cotangent of an angle. Remember the cotangent? It’s the inverse of the tangent, and is simply the length of the side adjacent to the angle (A) divided by the length of the side opposite the angle (A). It’s expressed as a ratio, fraction or decimal.
All the architect or builder would need would be the adjacent — the length of the base of the pyramid (half of it, actually, to make a triangle) and the opposite – the height at the center top. This would give the cotangent, which he could look up in the tables to find the angle. Or if he knew the angle he wanted, he could determine the ratio of bottom to height using the tables, and scale that to any size pyramid he wanted to build.
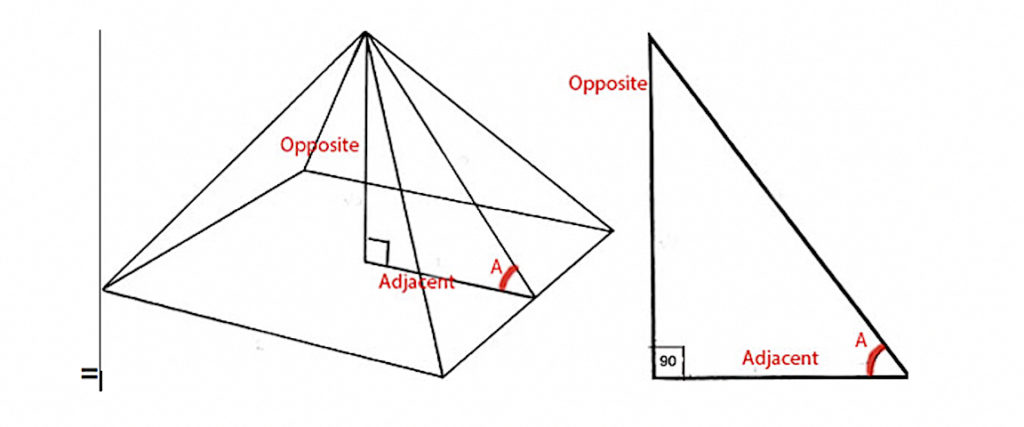
So, looking at the outer angle at A, if the base adjacent to the angle in question was 8 units, and the height, opposite our angle was 12 units, the cotangent is 8/12 or .6667. Just look it up in the table and voila, the angle of the outer stones would be between 56 and 57 degrees. But that’s not terrifically precise, so you’d use some math and iterative methods to come up with the exact degree – and I forget exactly how we did that in high school.
Better yet – if he had a calculator or PC, he would enter the cotangent as a decimal, .6667, in the lower box, and come up with 56.31 degrees for the outer angle. https://www.inchcalculator.com/cotangent-calculator/
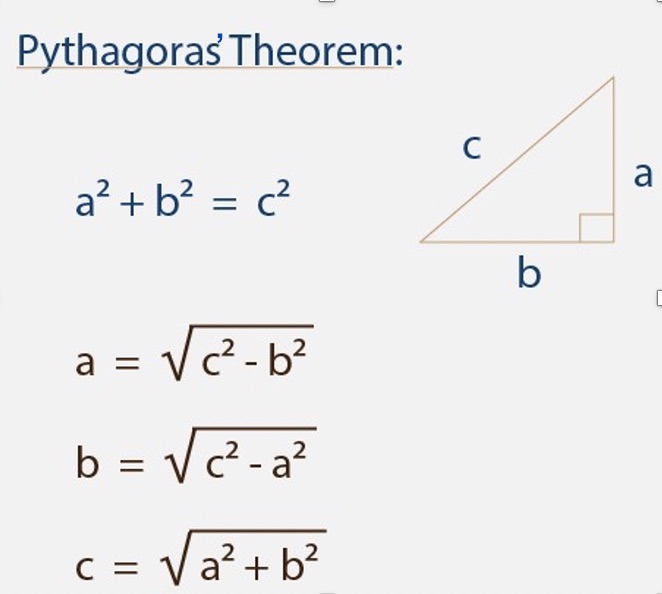
There’s another way they could figure out how long the sides of his pyramid would be. If Pythagoras had been around two thousand years earlier, came to Egypt and explained to Imhotep or Ankhhaf what a right triangle is and the mathematical relation between the sides and the diagonal, Ankhhaf could figure the length of any third side of his pyramid. It’s easy to figure out in your head if your triangle has a 3-4-5 shape: with a base of 4 units and a height of 3 units the hypotenuse is 5 units.
It gets a bit trickier with other dimensions that aren’t in those exact proportions. So in our triangle that’s 8 units long and 12 units high, you’d take 12 2 + 8 2 = 208, and then take the square root of 208. Help! In math classes you’d use something called prime factorization or do some fancy long division or an iterative method. The hypotenuse comes out to 14.42 units.
A calculator now gives you the hypotenuse, angles and measurements – just plug in the 8 and the 12, and it solves for all the unknowns. The exact hypotenuse is 14.42221 units. https://www.rapidtables.com/calc/math/trigonometry-calculator.html
There’s another critical measurement, the slope, how fast the triangle rises per unit of the bottom (“rise over run”). Our triangle rises 12 units for every 8, or 3/2, which is a slope of 1.5. And that is the same as the modern tangent (opposite over adjacent).
Ankhhaf (below) was Khufu’s half-brother, a prince and vizier-overseer of construction of the Great Pyramid at Giza. How did Ankhhaf and all the builders over the millennia make their calculations in order to cut the stones so perfectly without trig tables, calculators or input from Pythagoras?
For units of measurement, the Egyptians worked in digits, palms and cubits instead of inches, feet and yards, or centimeters and meters. Four digits (width of the finger) = one palm (the width of the palm), and 7 palms = one cubit (20.7 inches, originally the length of the Pharaoh’s forearm). And in the Rhind Mathematical Papyrus in the British Museum, copied around 1550 BC likely from a 250-year-earlier document, we learn of the seked. The seked is based on the ratio of the base to the height of a right triangle, which we know is, in fact, our modern cotangent.
The seked was expressed in cubits, palms and digits. It’s not actually the ratio of cubits to cubits; rather, it’s cubits, palms and digits mixed. To calculate, you divide 7 (the number of palms per cubit) by the slope (the rise over the run, or m). That number also happens to be 7 times the modern cotangent. In our triangle, which has a slope of 3/2 (or 1.5), 7 divided by 1.5 equals 4.66, the seked. Or we could take 7 times the cotangent, 7 X .6667, to get the same result. We’re doing math just like the ancient Egyptian pyramid builders!
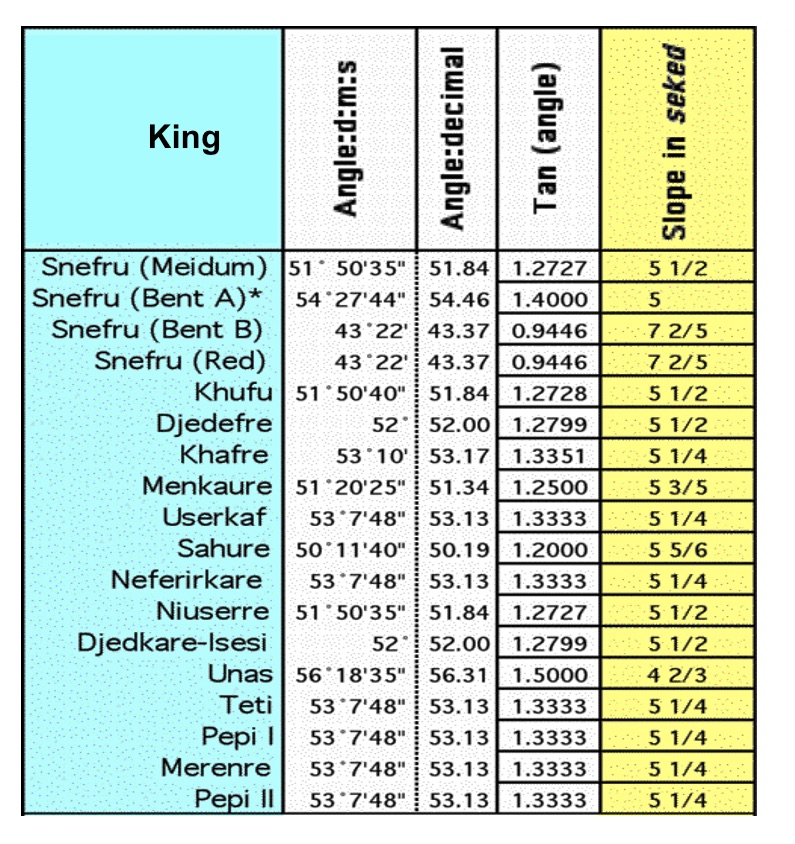
We can then find the angle with our calculator; Ankhhaf couldn’t do that. The table linked below shows the degrees of various angles associated with each seked, these calculated nearly four and a half millennia after the fact. (http://www.davidfurlong.co.uk/sekes0.htm). Ankhhaf’s Great Pyramid of Giza for Khufu was determined to have a seked of 5 ½, or 5 palms and 2 digits, which equals a slope of 1.27, with an outer angle of 51.84° The seked of the pyramid for Khefre (Chephren), Khufu’s son, was 5 ¼, or 5 palms and 1 digit, with an outer angle of 53.13°. The next table below shows the angles, tangents (slope) and sekeds for a number of pyramids. (https://egypt.hitchins.net/pyramid-myths/pyramids-have-different.html)
It’s also intriguing that the degree of the entrance passage to the Great Pyramid is 26 o 31’, which is a slope of extremely close to 1:2, and a seked of 14, used for most internal passages.
But all this still begs the question of what their precise level of mathematical understanding was to be able to design and build the pyramids. The Egyptians certainly understood a slope (our tangent), and the seked gave them the slope. They did not measure degrees of an angle, however; we determine those after the fact.
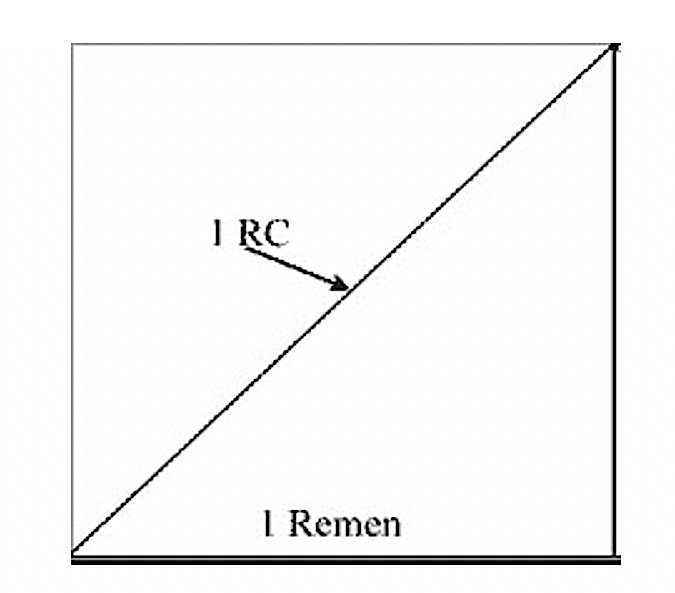
They may have understood the concept of a hypotenuse and its relationship to the other sides of a triangle: they had a unit of measure for land surveying called a Remen. The sides of a square are Remens and the diagonal, the hypotenuse, is the Royal Cubit.
It seems they were familiar with square roots, as math problems in both the Rhind Papyrus and the Lahun Mathematical Papyri ask questions about finding lengths of sides of a rectangle given ratios of the lengths. There is a hieroglyph for a square root; the word is kenbet, but we don’t know how square roots were calculated. They may have had tables with square roots, although none have been found.
There is debate as to whether the early Egyptians understood the theory behind the Pythagorean Theorem. It is likely, however, they knew the 3:4:5 ratio of a right triangle with its associated angles. Khafre’s pyramid has an angle of 53.13o which is a seked of 5.25, and the proportions for a triangle with this seked are 3:4:5. They certainly would have figured out that any right triangle, or pyramid, with these proportions is associated with that seked and that slope. It’s also interesting that Pythagoras was reported to have studied philosophy and mathematical science in Egypt!
Ankhhaf could figure out the slope (the tangent) without any tables or calculators, using rope, straight-edges and markings, and the seked. Builders and cutters drew on the side of the rectangular limestone block the dimensions of the height and bottom, which they determined from the seked. Then using a string or straight-edge, they connected the ends to create the diagonal. They then marked the stone, and the cutters chiseled away along the marked sloped line, likely with a copper blade. After polishing with sand and harder stone, the cut limestone block was ready to move from the quarry on the other side of the Nile to the site at Giza. (Local Giza fossiliferous limestone was from nearby a short distance south on the plateau; Fine Tura limestone from east of the Delta was used for outer casing in the Great Pyramid, angled precisely, while the interior was constructed with rough square and rectangular blocks of lesser quality local Giza limestone mortared together.)
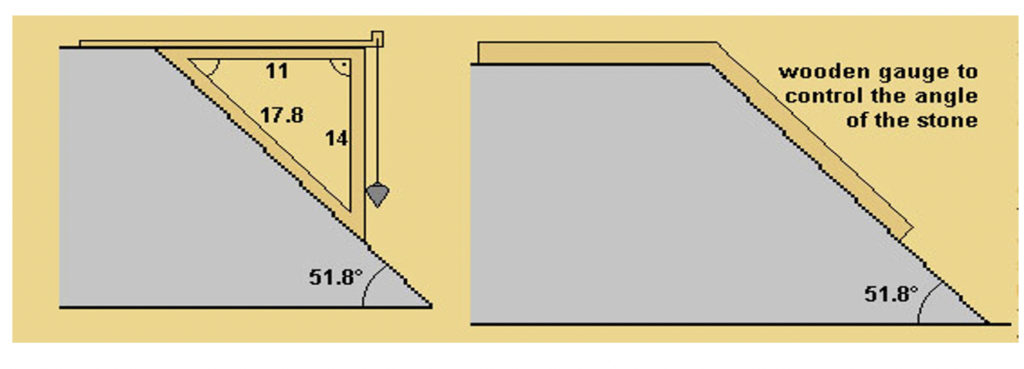
Since they understood ratios and could calculate measurements from sekeds, and the reverse, the measurements could be scaled up or down according to the dimension of stone needed for any given level of the pyramid. The pictures below show modern workers using Khufu’s pyramid’s dimensions of 11 along the bottom and 14 to the top, which happens to give an angle of 51.8o, and is a seked of 5½. These guys had cut this block from the quarry at Tura, took it across the Nile on a boat they built based on paintings in tombs, and used a sled, wooden rails and rollers to move it to this location.


Additionally, a combined level-square tool was used to maintain the precise and constant slope as they built from the ground up, and to make the four slanted edges form 90o angled corners horizontally. The two feet of the tool rest on the desired slanted edge, and where the plumb-bob falls is marked on a cross-bar. The seked was associated with the locations along the cross-bar. The right angles of the corners of the square base of the pyramid would be determined by laying the tool flat. (https://mae.ufl.edu/~uhk/EGYPTIAN-LEVEL.pdf)\
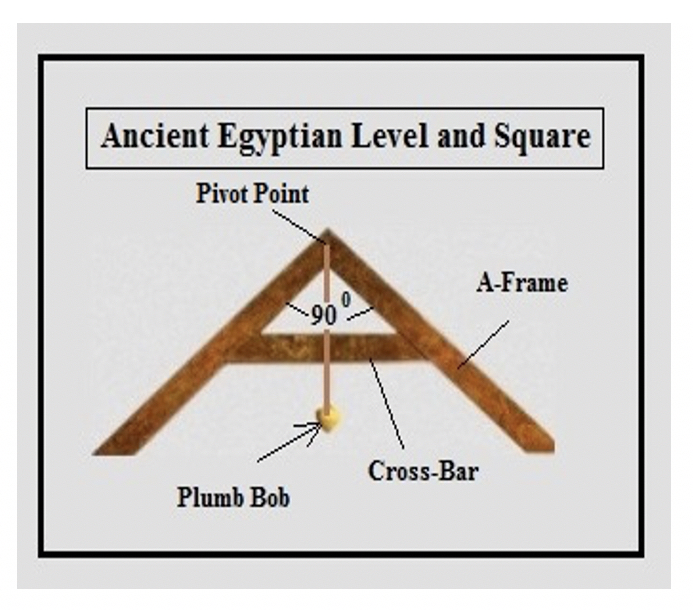
Other wooden guide-frames were also employed to keep the slope and angle constant. These below show the dimensions of Khufu’s pyramid.
So, while technically they weren’t using degrees, the Pythagorean Theorem or trigonometry, the Egyptians managed to cut their stones, size them according to placement, and build pyramids with consistent dimensions, slopes and angles (well, except for the Bent Pyramid, which starts at 54o and changes to 43o – trig tables might have helped the Ancient Egyptians, but these would be a long time in the future. Nonetheless, Ancient Egypt did well with what trigonometry it had by the Old Kingdom, despite transitional experiments like Sneferu’s Bent Pyramid at Dashur (ca. 2600 BCE) and the collapsed pyramid at Meidum possibly begun a little before the Bent Pyramid, both ca. 3rd Dynasty, before success at Giza in the 4th Dynasty about a century later.